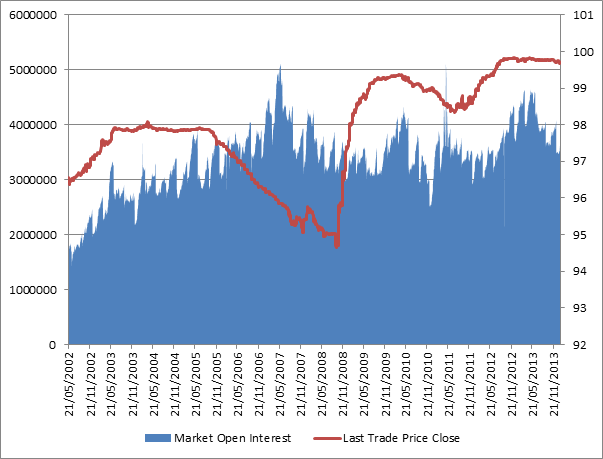
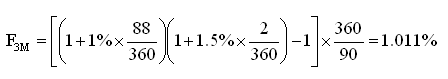The year-end effect in STIR futures is a legacy from the 1980’s. There are often high borrowing requirements at year end as Banks look to bolster their cash reserves at the end of a fiscal year or quarterly period. This requirement and the fact that the days straddling the end of one year and the beginning of the next fall in the holiday season and create an overnight borrowing period that can be two or four days in duration due to bank holidays.
In December 2013, the year end turn effect would have been muted since money could have been borrowed on Tuesday 31st December 2013 and returned on Thursday 2nd January 2014. This would have been just two days but if those days had straddled a weekend, it could have been four. It can have an effect on STIR futures because year end is included in the forward period covered by the December contract.
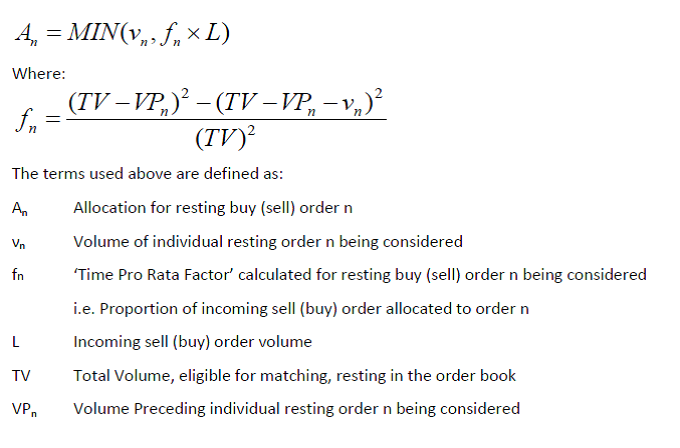
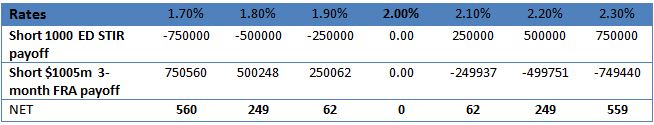
If the forward deposit period rate was 1%, then the futures implied rate should be
However, if rates jumped to 1.5% over the turn, the futures implied rate would be:
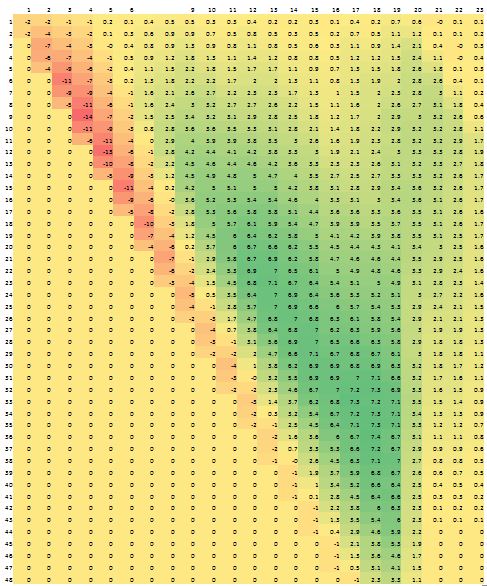
Clearly this is insignificant when the turn premium is 0.50% and the turn period two days so it is off most STIR trader’s radar apart from the most exceptional periods like run up to December 1999 when the markets were spooked by the millennium bug (Y2K) fears. This was most clearly observed in the Sterling Dec butterfly.
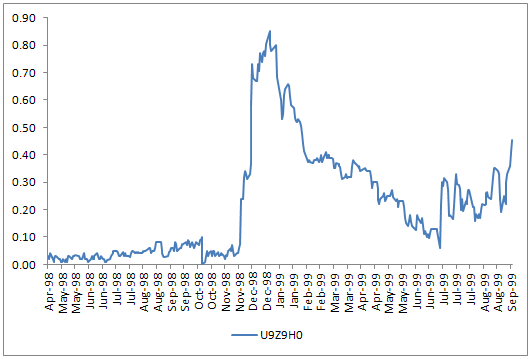
LIFFE Short Sterling U9Z9H0 butterfly Apr 98 to Sep 99 (price differential)
Worries that that non Y2K compliant Banks would be refused year end funding caused the Z99 future to fall sharply relative to the surrounding U99 and H0 contracts, causing the usually staid butterfly spread to blow out by around 80 ticks (bps). This also happened to Eurodollar and Euribor and was probably a once in a generation occurrence but watch out for potential repeats driven by, for instance, rumours of Banks failing regulatory requirements.
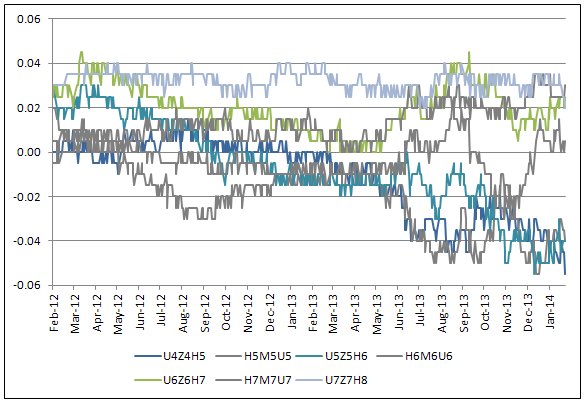
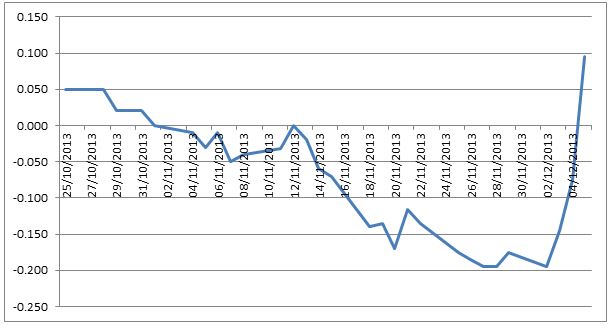
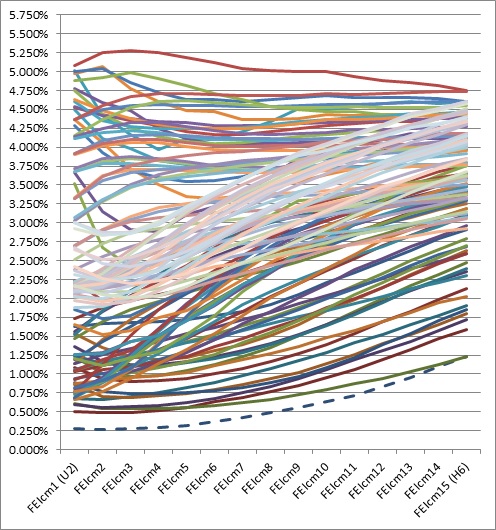
Looking at the latest set of Euribor Dec butterfly’s in the chart below, there are no discernible Dec premium reflecting year-end turn premiums, mainly because Central Banks are very adept at financing these days and post the financial crisis, Banks have relatively easy access to central bank funding
LIFFE Euribor Butterfly’s (Dec fly’s are coloured and surrounding fly’s are greyed for contrast)
However, despite the fact that the year-end turn effect seems to be diluted by the relatively small turn premiums in Dec fly’s these days, there is another aspect to consider relating to year end liquidity:
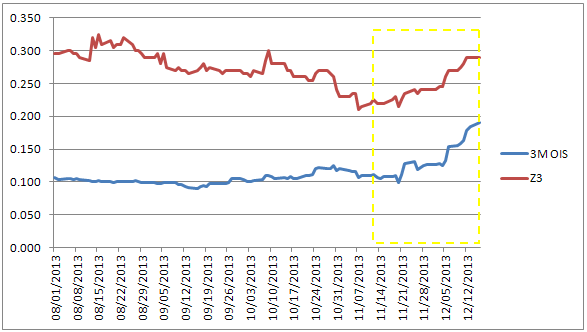
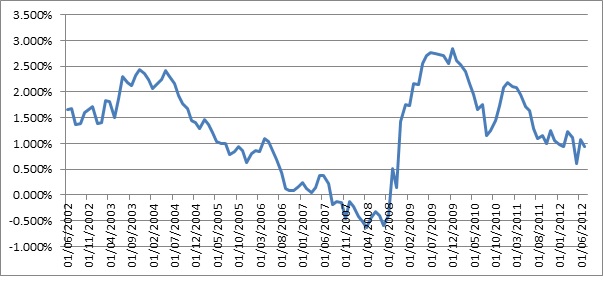
Stricter liquidity regulation caused a significant reduction in the liquidity available in the overnight market during December 2013, which fuelled sharp upward pressure on the overnight cost of cash. Having started December at 11.2bp, EONIA moved up to 20.6bp on 17 December, then declined a little to 17.1bp on 24 December before spiking in the last week of the year to 44bp in an illiquid market.
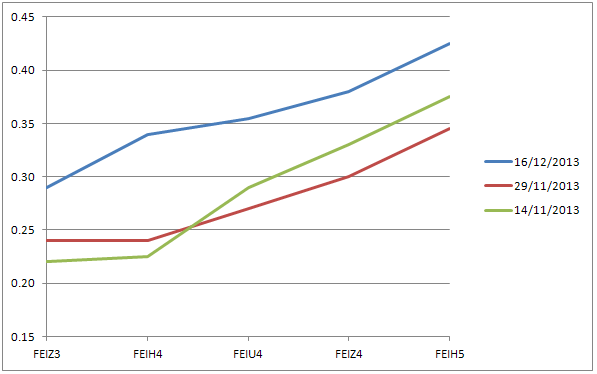
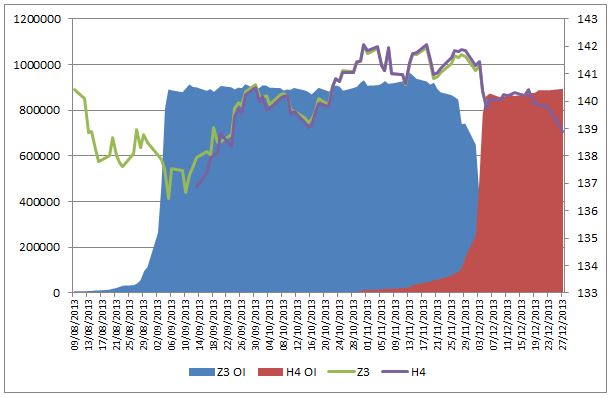
This effect can be observed on changes in the Euribor strip.
LIFFE Euribor Strips November to December 2013
The strip yield increases, almost in parallel as condition tighten in the EONIA market during December and the causal link is observable between 3M OIS and Euribor Z3.
Indeed, there seems to be a general pattern of tighter overnight rates in the euro zone and a quick study reveals that in seven out of the last ten years (2004 to 2013), Euribor futures closed lower (rates higher ) at the end of December compared to where they started December.